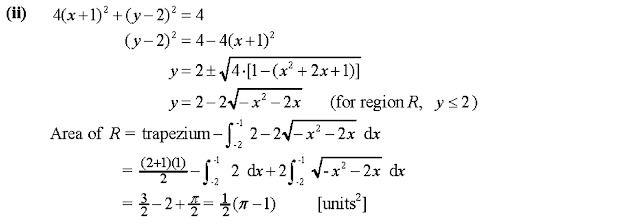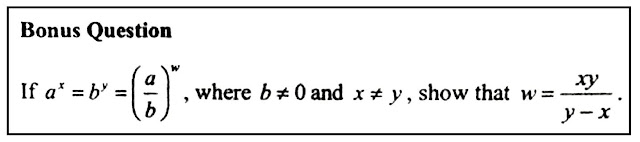[PriOlym_20151130RTAC] Ratio with One Circle Overlapping Two

Question

Introduction

     This question is like this previous one, except it is of olympiad standard.  I illustrate the solution of this without algebra, by using Distinguised Ratio Units.  As before, I try to match parts to an equal number.  But here we have quite a mixture of different types of units.

Solution

Commentary

     Basically we make the triangle units to number 12 and do the same for the circle and square units.  It turns out that one triangle unit is the sum of one circle unit and square unit.  We deduce that 9 circle units (for the area of A) plus 6 circle units (for the area of B) is the same as 8 circle units and 8 circle units.  The reduction of circle units must be equally compensated by the increase in the circle units.  Thus one circle unit is the same as two square units.  From here, things become easy.

Heuristics Used

H02. Use a diagram / model

H04. Look for pattern(s)

H05. Work backwards

H09. Restate the problem in another way

H11. Solve part of the problem

Suitable Levels

* Primary School Olympiad Mathematics

* Primary School Mathematics (challenge)

* other syllabuses that involve areas and ratios

* anyone who is game for a challenge

[AM_20151130IAXS] A Motif for the Absolutely Absolute

Problem

Introduction

     This question would pose a challenge for many students, although theoretically it is within reach of a good Additional Mathematics student (~ grade 10).  Graphs of both  sin x  and  cos x  are waves that oscillate up and down.  There are many pairs of vertical bars, indicating the absolute values or modulus, and these seem confusing.

Strategy

     Let us graph the functions  y = |cos x|   and  y = |sin x|.   Note that  ||sin x| – |cos x|| = ||cos x| – |sin x||.   The absolute difference of  |cos x|   and  |sin x|  is the difference between them ignoring the negative sign (if any) of the result.  And this is just the difference between the higher value and the lower value. 

Can you see any repeating patterns?  [H04]  Can you visualise the required area?  How many times is that of the basic pattern (known as “motif” in art)?  [H09, H10, H11]

Solution

Remarks

     Our total area is made up of four congruent pieces.  When  0 < x < ^p/₄,  cos x  is higher than  sin x.  That allows us to strip away all the absolute signs and do the calculation.

Heuristics Used

H04. Look for pattern(s)

H09. Restate the problem in another way

H10. Simplify the problem

H11. Solve part of the problem

Suitable Levels

* challenge for GCE ‘O’ Additional Mathematics  * IB Mathematics SL HL

* GCE ‘A’ Level H2 Mathematics  * IB Mathematics HL

* AP Calculus AB & BC

* University / College calculus

* other syllabuses that involve integration and area

* whoever is game for a challenge in integration

[H220151129IAAV] Integration for an Eclipsed Ellipse

Problem

Introduction

     Here we have a challenging H2 Mathematics question that tests students on the technique of substitution, finding area and volume of solid of revolution.  Note that the diagram is not drawn to scale and the line actually intersects the ellipse (oval shape) at the point (-2, 2).  Also the region  R  is the shaded area, but the label “R” is put outside of it.  Students should do their due diligence in ascertaining the intersection point themselves.

Solution

Remarks

     Since the word “exact” is not used in the instructions to part (iii), one can also use the Graphing Calculator to obtain the approximate answer  3.14  to 3 significant figures.  Part (iii) can also be done by another method of integration called the Shell Method, but this is not in the H2 syllabus.  Nevertheless, the student who uses it would not be penalised, unless explicitly forbidden in the rubric.

     The word “ellipse” means an oval shape, and is not to be confused with “eclipse” which means to occlude or hide (e.g. eclipse of the sun, eclipse of the moon).  By the way, the moon orbits around the earth in an ellipse and planets revolve around the sun in ellipses (ovals).
     Although not part of the syllabus, it may be advantageous to know that the area of a right ellipse is  pab,  where  a  and  b  are the semi-axes.  Imagine a circle of radius  a  being stretched by  ^b/_a.  Then its area  pa²  will be multiplied by the same factor  ^b/_a.  So this is not too difficult actually.  With the formula, one can verify one’s answer obtained by integration.

Heuristics Used

H02. Use a diagram / model

H04. Look for pattern(s)

H05. Work backwards        [ e.g. calculating new limits for substitution ]

H09. Restate the problem in another way

H10. Simplify the problem

H11. Solve part of the problem

Suitable Levels

* GCE ‘A’ Level H2 Mathematics

* IB Mathematics HL

* AP Calculus BC

* University / College calculus

* other syllabuses that involve applications of integration

* whoever is interested

[EM20151129CGCB] Bisector of a Chord in a Circle

Problem

Introduction

     This “elementary” mathematics question poses a challenge because it actually testing Coordinate Geometry and Circle Geometry.  In the setting of tests and exams, there seems to be a trend of combining topics.  To solve this problem successfully, students need to know that when a chord is bisected (cut into two equal parts), the line segment joining its mid-point to the centre of the circle will be perpendicular to the chord itself.  Thereafter, we can proceed with Pythagoras’ Theorem.

Solution

Remarks

     There is actually no boundary between topics and even subjects.  Things to be learned are separated into topics only to facilitate teaching of the material.  Students are encourage to adopt a more wholistic view of knowledge.

Heuristics Used

H04. Look for pattern(s)

H05. Work backwards

H09. Restate the problem in another way

H10. Simplify the problem

H11. Solve part of the problem

H13* Use Equation / write a Mathematical Sentence

Suitable Levels

* GCE ‘O’ Level Mathematics (“Elementary Mathematics”)

* GCE ‘O’ Level Additional Mathematics (revision)

* other syllabuses that involve geometry and coordinate geometry / analytic geometry
* whoever is interested

[Pri20151129RTAO] Equalising Ratio Units for The Overlap

Question

 

Introduction

     This is a primary school ratio problem that is quite a favourite among question setters, but poses headaches for pupils and parents.  The trouble is that the ratios use different base units and this makes it difficult to compare the ratios.  Can we avoid using algebra or trial and error?  

Strategy

     Note [H04, H09] that the difference in the areas between the rectangle and the square (including the shaded overlapping part) is exactly the same as the difference between them without the overlapping part.  With this crucial observation, we can proceed to try to equalise the ratio units [H10] of the aforementioned differences.  This can be done by multiplying to get to the Lowest Common Multiple, which, in this example is 6.  Henceforth we can be sure of using the same ratio units, because the same number of units are used to refer to the same quantity.

Solution

Summary

     Ratio problems are solved by making sure that we use the same type of units.

Heuristics Used

H02. Use a diagram / model        [ table ]

H04. Look for pattern(s)

H05. Work backwards

H09. Restate the problem in another way

H10. Simplify the problem

H11. Solve part of the problem

Suitable Levels

* Primary School Mathematics

* other syllabuses that involve areas and ratios

* anyone who wants to learn

[AM_20151127DTCR] The “Onion” Method for Differentiation

Question

Introduction

     The differentiation of the secant function is not taught directly as part of the Additional Mathematics syllabus.  It can be derived from known facts.  I first show the standard application of the (extended) Chain Rule for novices, and then show a more effective way of applying the chain rule, which I called the “Onion Method”.  This looks like peeling onions or unpacking Matryoshka dolls (“Russian dolls”). 

Reminders

Solution 1  (for beginners)

Solution 2  (a more expedient way)

Final Remarks

     When we peel onions, we peel from the outer layer inwards.  Likewise, when we have a composite function, we differentiate from the outer layer first, and then work to the inner layers.  Every time we differentiate a layer, we write down the changed layer and then copy and paste everything within that layer.  With regular practice, this should become second nature.
     For another example of the “onion”, take a look at the derivative of the arcsecant function.

Heuristics Used

H04. Look for pattern(s)

H05. Work backwards

H09. Restate the problem in another way

H10. Simplify the problem

H11. Solve part of the problem

H13* Use Equation / write a Mathematical Sentence

Suitable Levels

* GCE ‘O’ Level Additional Mathematics

* GCE ‘A’ Level H1 Mathematics

* GCE ‘A’ Level H2 Mathematics (revision)

* International Baccalaureate SL & HL Mathematics

* AP Calculus AB & BC

* other syllabuses that calculus

* anyone who loves to learn!

[AM_20151127DF2D] Differentiation with Chunking and Elimination

Question

Introduction

     Although this looks like a differential equation question, the student is not required to solve the differential equation.  The requirement is just to derive the equation.  This would be a challenging question for secondary 4 (~ grade 10) students taking Additional Mathematics or their counterparts in Integrated Programme schools.

Strategy

     One way to do this is to differentiate the given equation once and again and just verify the equation by substitution.  The problem is that when we repeatedly apply the Product rule

the terms tend to sprawl.  A way to keep things neat is to try to recognise chunks and also use elimination.

Solution

Remarks

     After differentiating once, we notice that  10xe^2x   is twice of  5xe^2x,  and this allows the simplification in [1].  The second differentiation yields  10e^2x   which, we notice, is twice of  5e^2x.  We can get rid of that term.   Multiplying equation [1] by 2 gives  10e^2x  in equation [3],  which matches nicely with the same term in  [2].  So we can eliminate that term via elimination.  After that, we just need to rearrange things to get the final equation.

Heuristics Used

H04. Look for pattern(s)

H05. Work backwards

H10. Simplify the problem

H11. Solve part of the problem

H13* Use Equation / write a Mathematical Sentence

Suitable Levels

* GCE ‘O’ Level Additional Mathematics, “Integrated Programme Mathematics”

* GCE ‘A’ Levels H2 Mathematics (revision)

* AP Calculus AB / BC (revision)

* University / College calculus (revision)

* other syllabuses that involve differentiation

* any learner interested in calculus

[H2_20151125DEST] Differential Equation via Substitution

Question

Introduction

     This question was taken from a Facebook group.  The suggested substitution was added in to make it accessible to students taking H2 Mathematics.  In the original question, no suggested substitution was given.

Strategy

     Observe that the given equation has  x  and  y.   After substitution, we should get an equation with  v  and  x only.  How to make  y  “disappear”?  One way is recognise patches that can be substituted for  v.  Another way is to make  y  the subject and differentiate that with respect to  x.  Replace the derivative ^dy/_dx  with your new expression.  Here, I do both at once!

Solution

Remarks

     Usually we try to express  y  in terms of  x,  but here, it is more convenient to express  x  in terms of  y.

     Since  A  is an arbitrary constant,  A+1  is still an arbitrary constant.  Also, whenever there is a “ln” appearing in the solution, it is good to introduce “ln” with an arbitrary constant.  So we can lump  A  and  1  together and call it  ln B.  Nothing is lost in this process.  ln B  is able to achieve all possible numbers.   ln B  is negative if  B  is a fraction between  0  and  1,  and is positive if  B  is more than  1  and is zero if  B = 1.

     This is not an applied differential equation, so there are no exogenic reasons or hints for us to suppose that  x + y + 2  is a positive quantity.  As such, we still need to leave it as | x + y + 2|.  To get rid of the absolute or modulus sign properly, we can replace  ±B  with  C.

Heuristics Used

H04. Look for pattern(s)

H05. Work backwards

H09. Restate the problem in another way

H10. Simplify the problem

H11. Solve part of the problem

H13. Use Equation / write a Mathematical Sentence

Heuristic that cannot be used here

H08. Make suppositions

Suitable Levels

*  GCE ‘A’ Level H2 Mathematics

*  IB HL Mathematics Calculus Option

*  Advanced Placement (AP) Calculus BC

*  university / college calculus

*  other syllabuses that involve differential equations

*  anyone who is game for a challenge

[S1_20151124AESR] Slanted Rectangle does not need Pythagoras

Question

Introduction

     This is another “Bonus Question” at a secondary level from somewhere that the question poser did not mention, but I guess it is most likely an Integrated Programme school in Singapore.  It is a beautifully crafted question.  The presence of a slant line seems to necessitate the usage of Pythagoras’ Theorem.  However, we have seen that Pythagoras’ Theorem can actually be avoided even in Primary (Elementary) School problems.  So a 10 year old kid with a rudimentary knowledge of algebra could do this.  Can you spot a short cut?

Making Observations

     Stare at the diagram for a while.  What do you observe?

Solution

             area of  DDB_nC_n =  ½  of the area of  AB_nC_nD.

              area of  DDB_nC_n =  ½  of the area of  DB_nPQ.

        \  area of DB_nPQ  =  area of AB_nC_nD = n cm².

HeuristicsUsed

H04. Look for pattern(s)

H05. Work backwards

H09. Restate the problem in another way

H11. Solve part of the problem

Suitable Levels

* Lower Secondary Mathematics

* challenge for Primary school Olympiad

* other syllabuses that involve areas and a tiny bit of algebra

* anyone game itching for a challenge

[H2_20151123APGP] Factor Theorem with Arithmetic and Geometric Progression

Question

Introduction

     This question tests students on their knowledge of arithmetic and geometric series.  They should also be familiar with Factor Theorem and methods of dealing with polynomials.  Once parts (i) and (ii) are solved, part (iii) is quite straightforward, provided that the student remembers how to deal with surds.

Review of Important Facts

Solution

Heuristics Used

H04. Look for pattern(s)

H05. Work backwards

H10. Simplify the problem

H11. Solve part of the problem

H13* Use Equation / write a Mathematical Sentence

Suitable Levels

* GCE ‘A’ Level H2 Mathematics

* IB HL Mathematics

* other syllabuses that series and Factor Theorem

[S2_20151122IXBQ] A Bonus for your Index Fun?

Question

Introduction

     This is a “bonus” question which most likely came from an Integrated Programme (IP) school.  It really tests the wits of students’ knowledge of indices and problem solving tactics.  I present two solutions without the use of logarithms.  The first solution uses reciprocal indices and the matching of the base ^a/_b.  In the second solution, we match up the indices to  xy.

Review of Important Laws of Indices

Solution 1

 

Solution 2

Remarks

     No logs from any forest were harmed in the process of making this blog post.  J

Heuristics Used

H04. Look for pattern(s)

H05. Work backwards

H09. Restate the problem in another way

H13* Use Equation / write a Mathematical Sentence

Suitable Levels

* challenge for Lower Secondary Mathematics (Secondary 2)

* GCE ‘O’ Level “Elementary” Mathematics (revision)

* other syllabuses that involve algebra and indices

[EM_20151120QGXS] Quadratic Graphs by Completing the Square

Question

Introduction

     This “Elementary” Mathematics question is usually pitched at secondary 3 (» grade 9).  It is quite a standard type of question, but from my experience, many students do not know how to begin.  The wording of the question (especially part (a)) may throw off some people.  The first stage in solving any mathematics question is always to make sure you understand the question, to know what is expected of you.  Once this is done, this question is actually quite straightforward if you know what to do and know where to look.  The coefficient of  x²  is  a = 1, so the question is not that tricky.  Part (a) is simply instructing you to complete the square.

Review

 

Solution

Tip:  You could get the y-intercept by using your fingers to cover the x² and x terms.  There is an even faster way: just look at the constant term!  You get the answer  5  immediately!

Heuristics Used

H02. Use a diagram / model

H04. Look for pattern(s)

H05. Work backwards

H09. Restate the problem in another way

H11. Solve part of the problem

Suitable Levels

* GCE ‘O’ Level “Elementary” Mathematics

* any syllabus that includes quadratic functions and parabolas

* anyone who is interested 

[AM_20151119] Anticipation and Bridging as Proof Tactics

Question

Introduction

     In a previous article, I have showed an “evil” tactic that can be used against “evil” questions.  Here is another “evil” trigonometric proof question.

     Many traditionalist school teachers insist on starting either from the LHS or the RHS, and working all the way to the other side.  Personally I do not mind any form of presentation as long as it is logical.  But not many students are able to do that.  People tend to fall into the trap of beginning a proof with the statement that they are supposed to prove in the first place.  This is called circular reasoning (or “begging the question” or petitio principii).  It is definitely a no-no.  So there is some advantage to sticking to the traditionalist mould.  The disadvantage is, of course, that it stifles creativity and this gives a misleading image of mathematics to the learner.

More “Evil” Tactics

     Now, if we do not want to “break the rules”, perhaps we can “bend the rules” a little.  On a piece of rough paper, or in your mind, secretly work from both sides and try to bridge them in the middle.  Let us compare

Think:  How are they similar?  How are they different?

As you can see, the LHS already has a preponderance of  cos 75°.  One of these  cos 75°  must somehow disappear.  The  RHS  has  4 sin 75°  which the LHS does not have.  So if we start from the LHS, we can use our magic wand [SV4] to create  4 sin 75°  out of thin air, remembering to divide by the same thing, so that the original value does not get changed.

Solution

Reflection

     Have you learned anything from this problem?  Let us review the heuristics used to help us solve this problem successfully.

Heuristics Used

H04. Look for pattern(s)

H05. Work backwards

H09. Restate the problem in another way

H10. Simplify the problem

H11. Solve part of the problem

Special Variants of Heuristics (Good for Trigonometric Proofs)

SV1   Work from both sides and try to bridge them in the middle [~ H04]

SV2   comparing (similarities/differences) what you have now with what you want [~ H05]

SV3   anticipate what will happen in the end and what you must do now [~ H05]

SV4   Magic Wand or create something out of nothing (无中生有) [~ H09]

Suitable Levels

* GCE ‘O’ Level Additional Mathematics

* IB SL & HL Mathematics (revision)

* other syllabuses that involve trigonometry

* just about anyone who is interested, really